Navigating Load Calculations for Modern Structures – Bridging Theory to Practice
Introduction
Understanding the various forces at play during structural building is essential. Every element of construction needs to be carefully analyzed for safety and efficiency, from the weight of the building materials to the dynamic pressures imposed by inhabitants and climatic conditions. These computations rely on several variables. Here is a guide for you all to understand the specifics of each sort of load and how we can handle it.
Calculating Different Structural Loads
Let’s consider a modern office building with a rectangular floor plan of 30 meters by 20 meters, consisting of 10 stories, with a floor height of 3.5 meters.
Dead Loads
During construction, dead loads are the total weight of all the materials used, such as beams, columns, walls, floors, and even cranes. To compute these static loads and get the overall weight per unit area, multiply the volume of each structural component by its unit weight. To ensure the stability and safety of the structure, this computation is crucial for sizing and strengthening structural elements.
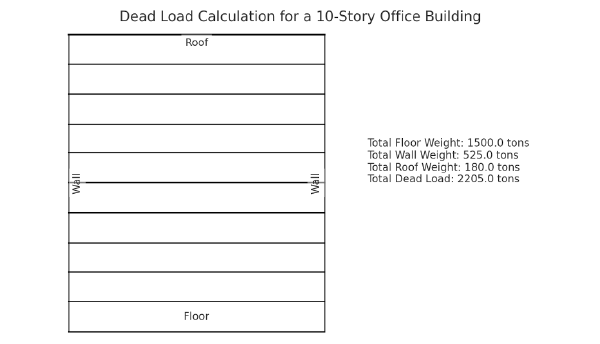
Dead Load Calculations for the above structure –
Dimensions and Specifications:
- Floor Area – 30 meters×20 meters=600 square meters
- Number of Floors – 10
- Floor Height – 3.5 meters
Weights per Square Meter:
- Floor Weight – 250 kg/m² (typical value)
- Wall Weight – 150 kg/m² (typical value)
- Roof Weight – 300 kg/m² (typical value)
Calculations
Total Floor Weight:
- Total Floor Weight = Floor Area×Weight per m² (Floor)×Number of Floors
- Total Floor Weight = 600 m²×250 kg/m²×10=1,500,000 kg=1500 tons
Total Wall Weight:
- Total Wall Weight = 2×(Length of Wall 1×Height+Length of Wall 2×Height)×Weight per m² (Wall)
- Total Wall Weight = 2×((30 m×10×3.5 m)+(20 m×10×3.5 m))×150 kg/m²
- Total Wall Weight = 2×(1050 m²+700 m²)×150 kg/m²=2×1750 m²×150 kg/m² = 525,000 kg = 525 tons
Total Roof Weight:
- Total Roof Weight = Floor Area×Weight per m² (Roof)
- Total Roof Weight = 600 m²×300 kg/m²=180,000 kg = 180 tons
Total Dead Load:
- Total Dead Load = Total Floor Weight+Total Wall Weight+Total Roof Weight
- Total Dead Load = 1500 tons+525 tons+180 tons = 2205 tons
So, the total Dead Load for the building is calculated to be 2205 tons. This calculation considers the weights of the floors, walls, and roof, using typical weight values for these components.
It’s crucial to remember that this computation counts on a steady demand throughout time.
Superimposed Dead Loads
Superimposed dead loads are additional, permanent ones introduced after construction, including MEP systems and moveable walls. Safety and integrity are guaranteed by precisely calculating and spreading their weight throughout the structure. To achieve precision, engineers need both manual computations and technology.
Superimposed dead loads include internal walls, floor screeding, floor finishes, ceiling materials, and MEPF (Mechanical, Electrical, Plumbing, and Fire Protection) lines and fittings in a typical residential concrete construction. To achieve correct load estimates, engineers may estimate a total weight per unit area, say 8 kN/m², considering each component’s density and thickness.
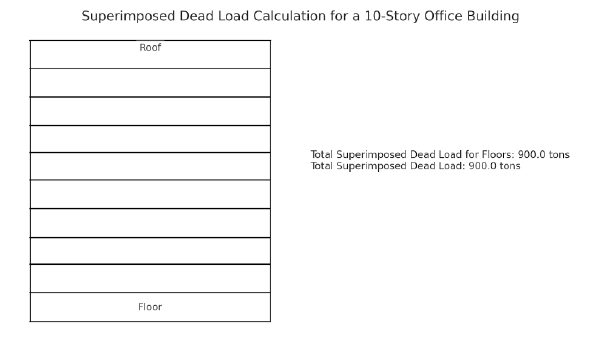
Superimposed Dead Load Calculations for the above structure –
Steps for Calculation
Assumptions for Superimposed Dead Load: Superimposed Dead Load per Floor – 1.5 kN/m² (typical value for office buildings)
Conversions –
1 kN = 100 kg
Calculations
Total Superimposed Dead Load for Floors –
- Superimposed Dead Load per Floor = Floor Area×Superimposed Dead Load per m² (Floors).
- Superimposed Dead Load per Floor = 600 m²×1.5 kN/m² = 900 kN = 900×100 kg = 90,000 kg.
- Total Superimposed Dead Load for 10 Floors = 90,000 kg×10 = 900,000 kg = 900 tons.
So, the total Superimposed Dead Load for the building is calculated to be 900 tons.
Live Loads
Live loads fluctuate according to the use and occupancy of a facility, excluding construction and external elements such as wind or snow. They include the weight carried by laborers, supplies, and inhabitants—vehicles, people, and furnishings—during the building process and its lifetime. They provide the stability and safety of the structure when combined with dead loads.
Building structural integrity and safety depend on precise live load calculations and their integration into the design process. For example, engineers have to take into account the weight of the people who live in the building as well as the equipment and furniture used in the offices.
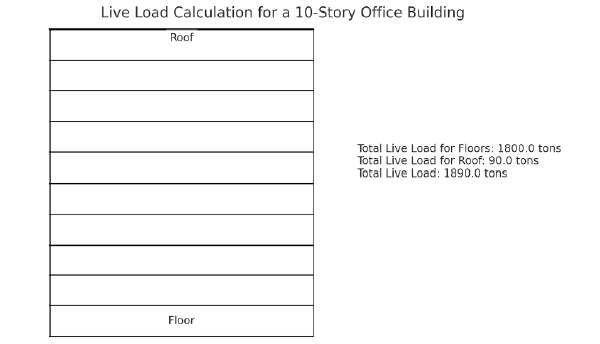
Live Loads calculations for the above structure –
Assumptions for Live Load: Live Load per Floor – 3 kN/m² (typical value for office buildings) Live Load on Roof – 1.5 kN/m² (typical value for roofs)
Conversions –
1 kN = 100 kg
Calculations
Total Live Load for Floors:
- Live Load per Floor = Floor Area×Live Load per m² (Floors)
- Live Load per Floor = 600 m²×3 kN/m² = 1800 kN = 1800×100 kg = 180,000 kg
- Total Live Load for 10 Floors = 180,000 kg×10 = 1,800,000 kg = 1800 tons
Total Live Load for Roof:
- Live Load on Roof = Floor Area×Live Load per m² (Roof)
- Live Load on Roof = 600 m²×1.5 kN/m² = 900 kN = 900×100 kg = 90,000 kg = 90 tons
Total Live Load:
- Total Live Load = Total Live Load for Floors+Total Live Load for Roof
- Total Live Load = 1800 tons+90 tons = 1890 tons
The total Live Load on the structure would be 1890 tons. Through precise consideration of these dynamic forces, structural engineers may create designs that uphold stability and safety in practical scenarios.
Seismic Loads
Building and bridge design in earthquake-prone locations must take seismic loads—the forces a structure endures during an earthquake—into consideration. To guarantee safety during an earthquake, these calculations take into account the degree of ground shaking, the kind of soil, the weight of the building, and dynamic properties. To get the accurate Seismic Load the mass is multiplied by its Seismic Coefficient.
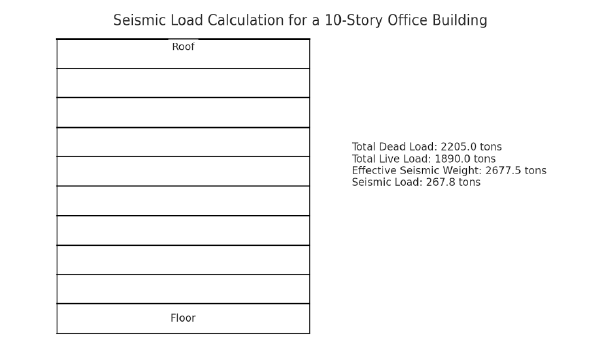
Seismic Load Calculations for the above structure –
Assumptions for Seismic Load:
- Seismic Weight – Typically includes the total dead load and a portion of the live load (usually around 25% of the live load).
- Seismic Coefficient – A value based on the building’s location, soil type, and design code (let’s assume a coefficient of 0.1 for this example).
Calculations
- Total Dead Load:
Total Dead Load = 2205 tons(from previous calculations)
- Total Live Load:
Total Live Load = 1890 tons(from previous calculations)
- Effective Seismic Weight (W):
𝑊 = Total Dead Load+0.25×Total Live Load
𝑊 = 2205 tons+0.25×1890 tons = 2205 tons+472.5 tons = 2677.5 tons
- Seismic Load (F):
𝐹 = 𝑊×Seismic Coefficient
𝐹 = 2677.5 tons×0.1 = 267.75 tons
So, the total Seismic Load on the structure would be 267.75 tons.
Wind Loads
Wind loads, or the forces the wind applies to structures, are important considerations in the construction of towers, bridges, and buildings. These loads are increased by taller buildings and higher wind speeds. When a building is properly designed for wind loads, it can resist these pressures, enhancing energy efficiency, preserving safety, and lowering maintenance costs throughout its lifetime.
Wind Load Calculations for the above structure –
Assumptions for Wind Load:
- Basic Wind Speed (V) – 50 m/s (typical value, may vary by location)
- Exposure Factor © – 0.85 (varies with building height and surroundings)
- Pressure Coefficient (Cp) – 1.3 (depends on building shape and orientation)
- Air Density (ρ) – 1.25 kg/m³ (standard value)
Wind Pressure (P)
𝑃 = 0.5×𝜌×𝑉2×𝐶×𝐶𝑝
𝑃 = 0.5×1.25 kg/m3×(50 m/s)2×0.85×1.3
𝑃 = 0.5×1.25×2500×0.85×1.3
𝑃 = 0.5×1.25×2500×1.105
𝑃 = 0.5×1.25×2762.5
P = 0.5×3453.125
𝑃 = 1726.5625 N/m2 = 1.73 kN/m2
Total Wind Load (F)
Area Exposed to Wind – Height of the building x Width of the building (10 stories x 3.5 meters/story x 30 meters)
Exposed Area = 35 meters×30 meters = 1050 m2
𝐹 = 𝑃×Exposed Area
𝐹 = 1.73 kN/m2×1050 m2 = 1816.875 kN
𝐹 = 1816.875 kN = 1816.875×100 kg = 181,687.5 kg = 181.69 tons
So, the total Wind Load on the structure will be 181.69 tons.
Slab Load Calculations
For structural design, it is essential to calculate the load on a slab, taking into account both the slab’s weight and additional loads like people and furniture. This procedure, called slab load calculation, guarantees building integrity and safety. To precisely evaluate these loads, engineers employ a variety of software tools, including STAAD-Pro, MBS, RISA, SAP2000, SAFE, and ETABS. Engineers can create structures that can resist the strains placed on beams and columns by figuring out how the slab distributes loads to them.
Slab Load Calculations for the above structure –
Steps for Slab Load Calculation Assumptions for Slab Load:
- Slab Thickness – 0.15 meters (typical value)
- Density of Reinforced Concrete – 25 kN/m³
Calculations
Slab Load (L)
Slab Load per m² = Slab Thickness×Density of Concrete
Slab Load per m² = 0.15 m×25 kN/m3 = 3.75 kN/m2
Total Slab Load for One Floor
Total Slab Load for One Floor = Floor Area×Slab Load per m²
Total Slab Load for One Floor = 600 m2×3.75 kN/m2=2250 kN
Total Slab Load for 10 Floors:
Total Slab Load for 10 Floors = Total Slab Load for One Floor×10
Total Slab Load for 10 Floors = 2250 kN×10 = 22500 kN = 22500×100 kg = 2,250,000 kg = 2250 tons.
The total Slab Load for 10 floors will be 2250 tons.
Beam Load Calculations
The forces that the beam can withstand are determined in beam load calculations. This includes figuring out how much the beam weighs in addition to any additional weights it has to support. In addition to any superimposed dead and live loads that could be applied to the beam, these extra loads also consist of the weight of the materials employed in the beam.
Factors for Beam Loads
- Beam Self-weight: Multiply the volume by the unit weight of the beam material to determine its weight. For example, the self-weight of a 300 x 450 mm concrete beam would be around 3.5 kN per meter.
- Superimposed Dead Loads: Take into consideration extra loads like the weight of the mechanical systems, the slab, and the walls. These are computed by the particular components that the system supports.
- Live Loads: Depending on how the structure will be used, take into account loads that may change over time. Based on the anticipated occupancy and activities within the structure, determine these loads.
Whether done manually or with the aid of software programs like STAAD-Pro, MBS, RISA, SAP2000, SAFE, and ETABS, structural calculations guarantee effective load distribution from slabs to supporting beams.
Beam Calculations for the above structure –
Steps for Beam Load Calculation
Assumptions for Beam Load:
Beam Spacing – 5 meters (typical value for an office building)
Beam Dimensions – 0.3 meters (width) x 0.6 meters (depth)
Density of Reinforced Concrete – 25 kN/m³
Calculations
Beam Volume per Meter Length:
Beam Volume per Meter Length = Width×Depth×Length per Meter
Beam Volume per Meter Length = 0.3 m×0.6 m×1 m = 0.18 m3/m
Beam Load per Meter Length:
Beam Load per Meter Length = Beam Volume per Meter Length×Density of Concrete
Beam Load per Meter Length = 0.18 m3/m×25 kN/m3 = 4.5 kN/m
Total Beam Length per Floor:
Total Beam Length per Floor = Floor Area/Beam Spacing
Total Beam Length per Floor = 600m2 / 5m = 120m
Total Beam Load for One Floor:
Total Beam Load for One Floor = Total Beam Length per Floor×Beam Load per Meter Length
Total Beam Load for One Floor = 120 m×4.5 kN/m=540 kN
Total Beam Load for 10 Floors:
Total Beam Load for 10 Floors = Total Beam Load for One Floor×10
Total Beam Load for 10 Floors = 540 kN×10 = 5400 kN
Total Beam Load for 10 Floors = 5400 kN÷9.81 ≈ 550.46 tons
The total Beam Load for the structure will be 550.46 tons
Column Load Calculations
Calculating column loads entails examining the forces acting on columns, taking into account both their weight and any extra loads.
Factors for column load calculation
- Column Self-Weight: Multiply the column’s volume by its unit weight to determine. Example: The self-weight of a concrete column with dimensions of 300 mm by 600 mm by 3 meters is around 1,338 kg.
- Superimposed Dead Loads: These are extra permanent loads originating from walls, slabs, and beams that the column bears. Only the items right above the column are subject to these loads.
- Live loads: Dynamic loads change over time and are determined by the structure’s intended use, taking into account things like equipment, furniture, and occupancy.
To estimate the sizes of structural elements, engineers employ software tools such as SAP2000, SAFE, ETABS, MBS, RISA, and STAAD-Pro in addition to manual computations. These instruments compute the axial load on each column by distributing loads from slabs and beams to columns. For instance, the way load is distributed to supporting columns in a two-way slab system is determined by the area of the slab that each column supports, or its tributary area. Precise load calculations guarantee that columns can securely support anticipated stresses, preserving structural soundness and security.
Column Load Calculations for the above structure –
Steps for Column Load Calculation
Assumptions for Column Load:
Column Spacing – 6 meters (typical value for an office building)
Column Dimensions – 0.4 meters (width) x 0.4 meters (depth)
Density of Reinforced Concrete – 25 kN/m³
Calculations
Column Volume per Meter Height:
Column Volume per Meter Height = Width×Depth×Height per Meter
Column Volume per Meter Height = 0.4 m×0.4 m×1 m=0.16 m3/m
Column Load per Meter Height:
Column Load per Meter Height = Column Volume per Meter Height×Density of Concrete
Column Load per Meter Height = 0.16 m3/m×25 kN/m3 = 4 kN/m
Total Column Height per Floor:
Total Column Height per Floor = Height of One Floor = 3.5 meters
Column Load per Floor:
Column Load per Floor = Column Load per Meter Height×Total Column Height per Floor
Column Load per Floor = 4 kN/m×3.5 m = 14 kN
Number of Columns per Floor:
Number of Columns per Floor = Floor Area/Column Spacing^2
Number of Columns per Floor = 600m2 /6m x 6m = 16.67 = 17 columns
Total Column Load for One Floor:
Total Column Load for One Floor = Column Load per Floor×Number of Columns per Floor
Total Column Load for One Floor = 14 kN×17 = 238 kN
Total Column Load for 10 Floors:
Total Column Load for 10 Floors = Total Column Load for One Floor×10
Total Column Load for 10 Floors = 238 kN×10 = 2380 kN
Total Column Load for 10 Floors = 2380 kN÷9.81 ≈ 242.45 tons
So, the total Column Load for the structure would be 242.45 tons.
Importance of Load Calculations
We all know that load calculations are important for varied reasons. Here are some of the key reasons for the importance of load calculations –
- Sizing Structural Elements: Finding the proper dimensions and strength for structural components such as slabs, columns, and beams is known as structural element sizing. It makes sure they can support weights without going above their limit.
- Ensuring Structural Stability: Accurate calculations ensure the structure’s strength. This takes into account active loads, which are momentary loads from people or equipment, dead loads, which are the weight of the structure, and environmental loads, which include wind and seismic stresses.
- Compliance with Safety Standards: Maximum load restrictions are established by building codes. Calculations guarantee that safety standards are met, avoiding collapse or deformation under anticipated loads.
- Optimizing Design: Design optimization is using the least amount of material possible to properly sustain loads. These optimizations produce economical and effective constructions.
- Avoiding Errors: Lowers the possibility of structural failures and assists in minimizing property damage and protecting public health.
Load calculations have a direct influence on the stability, economy, and safety of buildings, promoting the design of strong, durable, and safe constructions.
Conclusion
To sum up, load calculations are essential to structural design since they guarantee the security, steadiness, and effectiveness of structures. Engineers may design strong and affordable structures by precisely measuring all structural parts, including beams and columns, and taking environmental, living, and dead loads into account. Precise load estimates in structural engineering are crucial for mistake prevention, compliance with safety regulations, and design optimization. In the end, these computations have a direct bearing on the economics, stability, and safety of buildings, encouraging the creation of robust, long-lasting, and secure structures that endure the test of time.










